A line of research:
COLLECTIVE BEINGS: The Dynamic Usage of Models
by Gianfranco Minati, Eliano Pessa
This research is devoted to the problems to be dealt with when trying to manage emergent behaviors in complex systems, and of a number of proposals advanced to solve them. To this regard, the main concept, around which all arguments revolve, is the one of Collective Being. The latter expression roughly denotes multiple systems in which each component can belong simultaneously to different subsystems. Typical instances are swarms, flocks, herds, but even crowds, social groups, sometimes firms, and maybe the human cognitive system itself. The study of Collective Beings is, of course, a matter of necessity when dealing with systems whose elements are agents, each one of which is able to perform some form of cognitive processing. Therefore, the subject of this research could be defined as “the study of emergent collective behaviors within assemblies of cognitive agents”.
Needless to say, such a topic is connected to a wide range of applications and can attract the attention of a large audience. It integrates the contributions from Artificial Life, Swarm Intelligence, Economic Theory, but even from Statistical Physics, Dynamical Systems Theory, and Cognitive Science. It concerns domains such as the organizational learning, the development of an ethical code, the design of autonomous robots, and knowledge management in the post-industrial society. Managers, Economists, Engineers, as well as Physicists, Biologists, and Psychologists, can take advantage of the findings obtained through the transdisciplinary effort underlying the study of Collective Beings.
It is important to clarify why the traditional tools of Systemics, borrowed from Dynamical Systems Theory, are unable to deal with the problems tied to emergent behaviors within complex systems and, chiefly, within Collective Beings. To this regard, leaving aside the details of a debate about the notion of ‘emergence’ (Pessa 2002), lasting for almost a century, we will remind that, according to a very useful classification put forward by Crutchfield in 1994, we can distinguish between three kinds of emergence:
1) intuitive (or naïve ) emergence, in which the attribute ‘emergent’ is merely a synonymous of ‘new, unexpected’;
2) pattern formation, in which the emergence consists in the occurrence of a complex behavioral pattern as a consequence of simple laws and/or suitable constraints; however, this pattern comes from an explicitation (generally not trivial) of the dynamics already contained from the starting, even if in an implicit way, within the laws and the constraints adopted; a typical example is given by the solution patterns arising from bifurcation phenomena in systems of differential equations;
3) intrinsic emergence, referring to the cases in which the occurrence of behavioral patterns, even if compatible with the laws and the constraints in use, cannot in principle be foreseen in advance only relying on these latter.
It is easy to understand how the notion of intrinsic emergence is more powerful than the notion of pattern formation. Namely, in the latter case we could always foresee the patterns arising as a consequence of a given law and/or of given constraints, provided we would be equipped with a sound mathematical theory (admittedly a not trivial requirement). The presence of such a theory, however, would not be enough for forecasting the patterns occurring in a situation of intrinsic emergence. In other words, whereas in the case of pattern formation the complexity consists only in making explicit information already present under an implicit format, in the case of intrinsic emergence the complexity lies in the very nature of the system itself.
As a consequence of these arguments, we are forced to rely only on the notion of intrinsic emergence when dealing with complex systems. To this regard, we must underline that the intrinsic emergence is associated to further features, the most prominent being the following ones:
The set of features we illustrated before gives rise to a number of strong constraints on the possible models allowing for intrinsic emergence. However, arguments related to mathematical convenience actually prevent from attempts to fulfill these constraints in a rigorous way within systems constituted by a finite (and fixed) number of components, lying within spatial domains of finite extension. Thus, the only solution so far adopted in order to build mathematical models of intrinsic emergence has been the one of resorting to descriptions working in an infinite volume limit, and based on Quantum Field Theory (QFT) (Vitiello 2002). Namely, on one hand, the classical deterministic models (such as the ones based on classical mechanics, or the models proposed within the framework of the so-called ‘Systems Dynamics’, introduced in early times by Forrester, and even the ‘dissipative structures’ studied by Prigogine and his school) can describe only pattern formation, their behaviors being always predictable, at least in principle; on the other hand, the models based on ordinary Quantum Mechanics can describe only systems containing a finite and fixed number of elementary components.
Within the framework of QFT the intrinsic emergence is described as a phenomenon of Spontaneous Symmetry Breaking (SSB), arising from the fact that a model parameter, while changing, crosses a critical value, and this produces an increase of the number of the possible ground states (that is stable equilibrium states associated to a global minimum of the energy). In such a situation the system must choose, between the available ground states, the one around which its future evolution will take place. On one hand, the outcome of this choice is, in principle, intrinsically unpredictable, as all ground states are, within the model, reciprocally equivalent. On the other hand, once made such a choice, it entails the birth of collective excitations, in turn responsible for long-range correlations (the so-called ‘Goldstone bosons’) preventing the system from a change, under the influence of perturbations, of the choice already made (a property which some people refer to as generalized rigidity).
Such a picture obtained a remarkable success in theoretical physics, and allowed for a complete explanation (together with experimental predictions) of spectacular phenomena such as superconductivity, superfluidity, ferromagnetism, laser effect, as well as of the elementary particle phenomenology, the unified theory of fundamental interactions, the birth and evolution of the Universe, and the theory of phase transitions. However, despite these achievements, such a description of the intrinsic emergence leaves unanswered a number of fundamental questions, such as:
a) do we really need the mathematics of QFT ? It is still unclear whether the intrinsically emergent effects, such as the ones exhibited by SSB within QFT, could or not occur even in systems of not-quantum nature; between these latter the most promising candidates are the stochastic systems, in which a traditional classical model is affected by some form of noise; to this regard, a number of results was already obtained, concerning the formal equivalence between some kinds of stochastic systems and suitable QFT models;
b) how to describe the boundaries? Real systems don’t work in an infinite volume limit; we therefore need a theory explaining how a boundary arises and evolves, as a byproduct of the interaction between a system and its environment; such a theory needs, in turn, suitable methods for describing effectively the environment itself; such a perspective was not taken seriously until recent times, and the description of the environment is actually a subject of deep investigation;
c) how to describe the intrinsic emergence in biological systems, or in systems composed by agents, each one endowed with a cognitive system ? Whereas a quantum field can be interpreted as a systems composed by a (variable) number of particles, each one belonging to a specific kind, interacting according to well-defined, and invariant laws, a biological as well as a cognitive, social, or economic system contains components which can play simultaneously different roles, and which interact in multiple fashions, in a way which can change from time to time; besides, the evolutions of such systems revolve around metastable local equilibrium states, very different from the ground states taken into consideration by QFT.
These questions must necessarily be dealt with if we undertake an analysis, and a synthesis (in engineering sense) of complex systems made by interacting cognitive agents. To this regard, in this research we introduce a new perspective which, while retaining the validity of the descriptions of intrinsic emergence proposed by theoretical physicists, tries to generalize them within a systemic framework. The attribute ‘systemic’ means that this framework fits within Systemics, a thinking movement which originated from General System Theory, proposed by Von Bertalanffy, and from Cybernetics, introduced by Wiener, and developed by Ashby and Von Foerster. A systemic framework is characterized by the following features:
Ø the focus is on the global, wholistic properties of entities qualified as systems, which, in general, are described in terms of elements and of their interactions;
Ø the role, and the nature of the observer are taken into account, as far as possible, within the description and the modelling of every phenomenon;
Ø the goal is not the one of obtaining the unique correct model of a given behavior, but rather of investigating the complementarity relationships existing between the different models of the same phenomenon.
In this research we consider, to better specify the domain under study, a distinction (which could be seen even as hierarchical) between different kinds of systems:
v simple systems, characterized by the fact that each component is associated (in an invariant way) to a single label (which can be even coded through a number), specifying the nature and the allowable operations of the component itself; a limiting case of simple systems is given by the sets, in which the single components cannot perform operations, but only exist; it is, however, possible to build models of intrinsic emergence based on suitable forms of simple systems: the models proposed by theoretical physicists belong just to such a category;
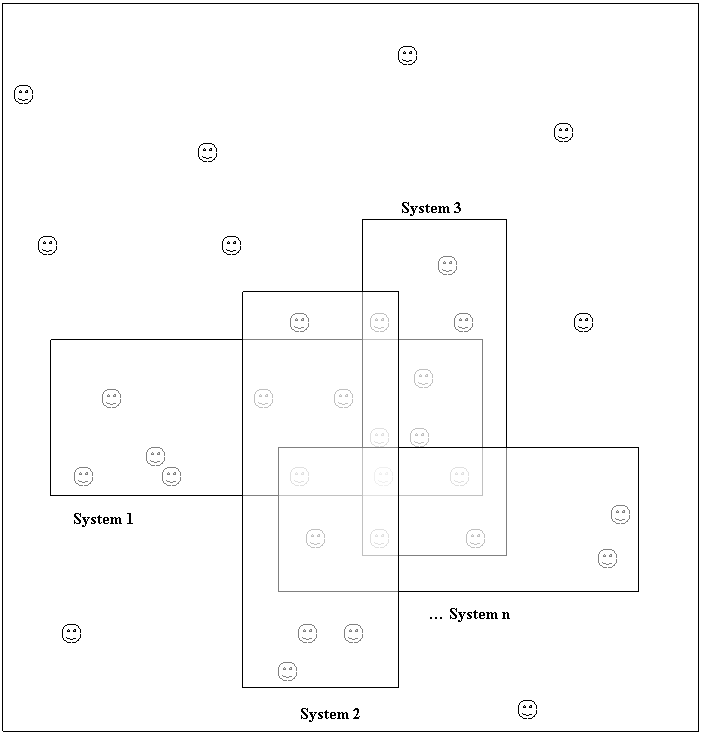
v collective beings (Minati 2001), characterized by the fact that each component is associated (in a variable way) to a set of possible labels; the association between the component and the labels depend on the global behavior of the system itself, and can vary with time; a typical case is offered by a flock of birds, in which each bird belonging to it can be associated to a single label, specifying both the relative position of the bird itself within the flock, and the fact that its operation consists only in flying in such a way as to keep constant its distance with respect to the neighboring birds; however, such an association holds as long as the flock behaves like a flock, that is like a single entity; as soon as the flock loses its identity, a single bird becomes associated to a set of different labels, specifying different possible operations, such as flying, hunting, nesting, and so on; this new association can define a different collective being, such as a bird community;
v multi-collective beings, characterized by the existence, not only of different components, but even of different levels of description and of operation; each component and each level is associated (in a variable way) to a set of possible labels; the forms of these associations depend on the relationships existing between the different levels; examples of multi-collective beings are offered by the human cognitive system, and by the human societies.
In this research we particularly consider the study of collective beings and, in addition to a review of the existing possibilities for modeling their behaviors, we introduce a general methodology for dealing with these complex systems: the dynamic usage of models (DYSAM) (Minati 2002a). The latter applies to the cases in which it is manifestly impossible, in principle, to fully describe a system through a single model. The main components of DYSAM are:
§ a repertoire of different possible models of the same system;
§ a strategy for selecting, as a function of the general and of the momentary goals, of the available knowledge, and of the context, the models to be used (and eventually integrated) to gain new knowledge about the system under study; such a strategy is not fixed but can vary with the evolution of the interactions between the observer and the system.
In applications of DYSAM methodology we mention the cases in which each model of a given complex system must necessarily include the observer as a part of the model itself. Such a circumstance is mandatory when dealing with complex cognitive, economic or social systems. Here DYSAM can reveal its usefulness, in helping to manage the problems arising from the interactions with these systems, as well as within them. This methodology is a powerful approach in order to implement strategies no more based on standardizing and averaging, but on multiple modeling.
With reference to quantitative modeling, in this research we focus on possible quantitative criteria for detecting emergence within a given system. To this regard, two categories of criteria are reviewed:
A. criteria for detecting any form of emergence;
B. criteria for detecting only intrinsic emergence.
Within the criteria belonging to the class A, the research focuses on a criterion never previously used in literature, based on a suitable measure of the variations of ergodicity of the system under study (Minati 2002b). As is well known, a system, described at a microscopic level as an assembly of mutually interacting elementary components, is ergodic when the average, at a single instant of time, on all microscopic behaviors present within the system, is equal to the time average on the behavior of a single component. The ergodic systems can be considered as the normal systems, on which it is possible to apply the traditional methods of statistical mechanics, in order to connect in a clear way the microscopic features with the macroscopic phenomenology revealed through the experimental observations. Besides, such a phenomenology consists in a relaxation towards a stable equilibrium state, in which the macroscopic features of the system can be observed with the minimum possible uncertainty. The property of ergodicity, of course, is completely lost during a phase transition, or a structural change. Thus, by detecting an increase of ergodicity (measured in a suitable way) within a system, we can recognize that this latter is evolving from a structural change towards a new form of equilibrium, which, rightly, can be considered as emergent from the previous state.
The methodology and the quantitative criteria used in this research allow for a unified approach to a number of problems dealt with in studying, modeling, managing complex systems. Between these problems a special place is deserved to the foundations of Cognitive Science, the Swarm Intelligence, the development of firms, the birth of an ethics within a group of individuals, the teaching and educational strategies, the design of ‘intelligent’ software. Of course, any new methodology solves some problems but, in turn, creates many new problems. The attempts to solve these latter, and the debate about the advantages and the shortcomings of a new proposal have always been the motor of any investigation and of any progress. We hope this research will offer a little contribution in this direction.
References
Minati G., Brahms S., (2002a), THE DYNAMIC USAGE OF MODELS (DYSAM), in Minati G., Pessa E., eds, (2002), Emergence in Complex Cognitive, Social and Biological Systems, Kluwer
Minati G., (2002b), EMERGENCE AND ERGODICITY: A LINE OF RESEARCH, in Minati G., Pessa E., eds, (2002), Emergence in Complex Cognitive, Social and Biological Systems, Kluwer
Pessa E., (2002), WHAT IS EMERGENCE?, in Minati G., Pessa E., eds, (2002), Emergence in Complex Cognitive, Social and Biological Systems, Kluwer
Vitiello G., (2002), QUANTUM FIELD THEORY AND SYSTEMS THEORY, in Minati G., Pessa E., eds, (2002), Emergence in Complex Cognitive, Social and Biological Systems, Kluwer
and from books and papers on Systemics
Minati G., ESSERI COLLETTIVI, Apogeo scientifica, 2001 (COLLECTIVE BEINGS, in progress)

Fig. 1 – A schema illustrating the emergence of a Collective Being (multiple systems) from same interacting agents